Welcome to my website!

The classification of (semi)simple Lie algebras over the field of complex numbers is regarded by many as a jewel of mathematics. It was first described by German mathematician Wilhelm Killing in a series of papers published between 1888-1890. A more rigorous proof (and the case of real Lie algebras) was presented by Élie Cartan in his 1894 PhD thesis. In 1947 the 22-year old Eugene Dynkin worked out a modern, streamlined proof of the classification theorem.

The theorem states that every semisimple complex Lie algebra is a "sum of building blocks", most of which belong to one of four infinite families. These are denoted by A$_n$, B$_n$, C$_n$, D$_n$, where $n$ is an arbitrary positive integer. Surprisingly, there exist five exceptional "building blocks" that don't fit into the above families. They are named E$_6$, E$_7$, E$_8$, F$_4$, G$_2$.

The classification theorem heavily relies on abstract mathematical objects called root systems, which are symmetric configurations of vectors (usually) sitting in higher dimensional space. The dimension of this space is indicated by the subscripts, e.g. E$_8$ lives in an $8$-dimensional Euclidean space.

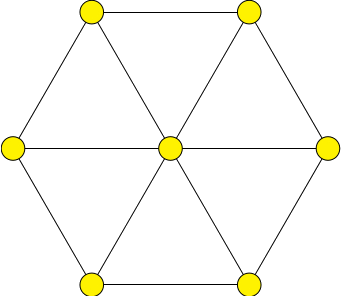
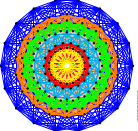
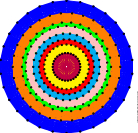
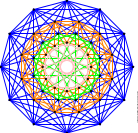
The frame of a cube can cast shadows of different shapes, but only a few orientations lead to the most symmetric shadows, namely the projections through one of the four body diagonals. See the image on the right. The method of finding the "most symmetric shadows" can be generalized to higher dimensions, and this is how the string arts of the exceptional root systems E$_6$, E$_7$, E$_8$, F$_4$, G$_2$ were made. The needles of the string arts point where the end points of root vectors land after projection.

The connections are obtained by connecting every vector with their nearest neighbours before the projection.$^\dagger$ A fun fact is that due to the left-right symmetry of the connections we have an even number of threads meeting at every needle, In graph theory such a structure is called an Eulerian Circuit. meaning that the connections with different colours can be drawn using a single, but really long piece of thread without cutting.$^\ddagger$

$^\dagger$ There are some extra connections in the case of G$_2$.
$^\ddagger$ There's only one exception. Can you guess where?